NASA is currently developing the Artemis mission to return people to the Moon after a 50-year hiatus. However, when we begin to explore the surface of our natural moon, the question arises: how will astronauts navigate there? To do this, we need a global navigation satellite system (GNSS). An 800-year-old mathematical trick can come to the rescue here. This mathematical approach is known as the Fibonacci sphere.

Researchers from Eotvos Lorand University in Hungary use this method to better estimate the ellipsoid of the Moon’s rotation — it got this shape due to gravitational interaction with the Earth. At first glance, it may seem that both of these objects are ideal spheres, as depicted in many illustrations of the Solar System. However, the influence of gravity, rotation and tidal fluctuations leads to the fact that they look more like flattened balls.
Geophysicist Gabor Timar and student Kamilla Cziráki, in their article published on Acta Geodaetica et Geophysica, write that due to the lesser degree of flattening of the Moon compared to the Earth, it would be better to use a spherical coordinate system there.
“GNSS technology uses a rough estimate of Earth’s squashed ball shape. If we’re to develop a Geographic Information System (GIS) for the lunar surface, we need the same estimate for the Moon’s selenoid (the equivalent of Earth’s geoid, or true, irregular shape). However, with the renaissance of lunar missions, it seems worthwhile to define an ellipsoid of revolution that better fits the selenoid,” explains Gábor Timár.
Moon and Fibonacci Sphere
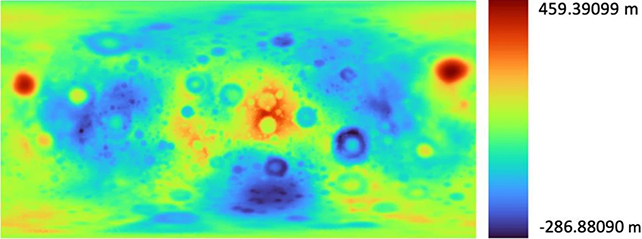
To do this, the researchers turn to the Fibonacci sphere, which uses an approach based on the Fibonacci sequence to evenly distribute points on the sphere. Chiraki and Timar applied a computational model to map 100,000 points of the moon’s surface using NASA measurements. Cziráki and Timar applied a computational model to map 100,000 points of the moon’s surface using NASA measurements.
This approach made it possible to obtain more accurate data for the major and minor axes defining the ellipsoid of the Moon’s rotation. In particular, the lunar poles are about half a kilometer closer to its center than the equator, and taking this information into account in any future lunar GPS will help reduce the number of incorrect definitions of coordinates on the Moon.
Such detailed calculations have not been carried out for the Moon since the 1960s. It is also worth noting that when the researchers applied their methodology to the Earth, the data obtained exactly coincided with the already known ones, which further confirmed the accuracy of the approach.
The results of this study will help to create more successful navigation systems for people who will go to the Moon in the future.
Earlier we reported on how artificial intelligence improved the formula for estimating the mass of galactic clusters.
Follow us on Twitter to get the most interesting space news in time
https://twitter.com/ust_magazine

